Here is the solution for previous question
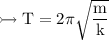
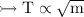
Hence
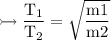
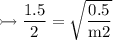

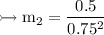
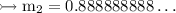
So
from this
So it's infinite in terms of 8 so must be greter than one 8
We rounded it up for 3 decimals
So
Error range=[1.99,2.01]
Very mere one
Now find percentage
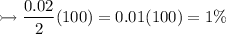
Mass should be lied in
But for increase mass
it would be
- 0.00889-0.00500=0.00389kg