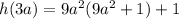Answer:
The values are
a)

b)
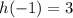
c)
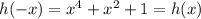
d)
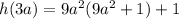
Explanation:
Given that the function h is defined by
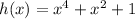
To find
a) h(3)
Put x=3 in the given function
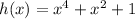 we get
we get

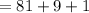
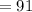
Therefore

b) h(-1)
Put x=-1 in the given function
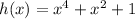 we get
we get
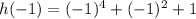
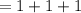
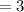
Therefore
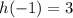
c) h(-x)
Put x=-x in the given function
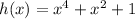 we get
we get
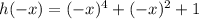

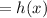
Therefore
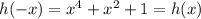
d) h(3a)
Put x=3a in the given function
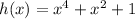 we get
we get
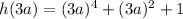
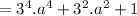
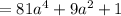
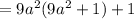
Therefore