Answer:
a) 1.75m/s
b) 14,062.5J
Step-by-step explanation:
- a) We solve this problem using linear momentum

where
 is the momentum,
is the momentum,
 is the mass, and
is the mass, and
 is the velocity.
is the velocity.
the momentum of the first train before the collision:
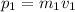
where
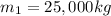 and
and

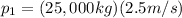
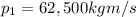
the momentum of the second train before the collision:
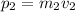
where
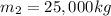 and
and
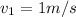

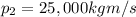
the total momentum before the collision is:
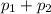
and due to the conservation of linear momentum: the amount of linear momentum before the collision must be the same after the collision.
so due to conservation:

the linear momentum of the two train system after the collision
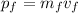
where
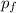 is the final linear momentum,
is the final linear momentum,
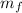 is the final mass of the system, if the two cars end up connected the mass is:
is the final mass of the system, if the two cars end up connected the mass is:
 (the sum of the mass of the two cars)
(the sum of the mass of the two cars)
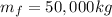
and
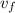 is the final speed: the speed of the connected cars.
is the final speed: the speed of the connected cars.
so, going back to the conservation of momentum
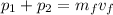
replacing all known values:
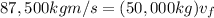
clearing for the final speed:
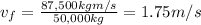
- b) the initial kinetic energy:
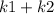
which is:
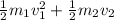
replacing all known values:

anf the final kinetic energy is:
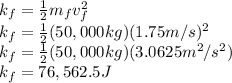
the difference is:
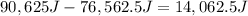
the kinetic energy of the system decreased 14,062.5J