Question

Consider the following system of inequalities below.

Line 1: 3y<2x+18

Line 2: -4y<-x+12

Which of the following points are in the solution set? Select all that apply.


a. (-4, -3)

b. (1, 6)

c. (2, 4)

d. (5, -5)

e. (3, 2)

Answer 1

Option A, B, C

(-4, -3) and (1, 6) and (2, 4) is a solution to given system of inequalities

Solution:

Given system of inequalities are:

Line 1 : 3y < 2x + 18

Line 2 : -4y < -x + 12

Let us substitute the given solution set in options and check if it satisfies both the inequalities

Option A

Substitute x = -4 and y = -3 in Line 1

`3(-3) < 2(-4) + 18\\\\-9<-8+18\\\\-9<10`

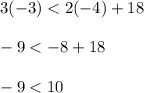
-9 less than 10 is true

Substitute x = -4 and y = -3 in Line 2

`-4(-3) < -(-4) + 12\\\\12<4+12\\\\12<16`

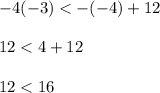
12 less than 16 is true

Thus (-4, -3) is a solution to given system of inequalities

Option B

Substitute x = 1 and y = 6 in line 1

`3(6)<2(1) + 18\\\\18<2+18\\\\18<20`

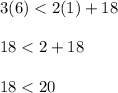
18 less than 20 is true

Substitute x = 1 and y = 6 in line 2

`-4(6) < -(1) + 12\\\\-24<-1+12\\\\-24<11`

-24 is less than 11 is true

Thus (1, 6) is a solution to given system of inequalities

Option C

Substitute x = 2 and y = 4 in line 1

`3(4) <2(2) + 18\\\\12<4+18\\\\12<22`

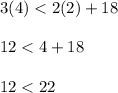
12 is less than 22 is true

Substitute x = 2 and y = 4 in line 2

`-4(4)<-(2) + 12\\\\-16<-2+12\\\\-16<10`

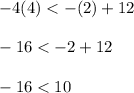
-16 less than 10 is true

So, (2, 4) is a solution to given system of inequalities

Option D

Substitute x = 5 and y = -5 in line 1

`3(-5) <2(5) +18\\\\-15<10+18\\\\-15<28`

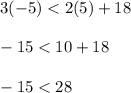
-15 is less than 28 is true

Substitute x = 5 and y = -5 in line 2

`-4(-5)<-(5) + 12\\\\20<-5+12\\\\20<7`

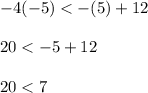
20 less than 7 is not true

Thus (5, -5) is not a solution to given system of inequalities

Option E

Substitute x = 3 and y = 2 in line 1

`3(2)<2(3)+18\\\\6<6+18\\\\6<24`

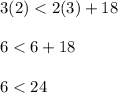
6 less than 24 is not true

Thus (3, 2) is not a solution to given system of inequalities