Question

A 1445-kg car,c, moving east at 21.2m/s, collides with a 2625-kg car,d,moving south at 17.5 m/s, and the cars stick together. In what direction and with what speed do they move after the collision

Answer 1

After the collision, they move with a speed of 13.57 m/s at 56.30° south of east

Step-by-step explanation:

Conservation Of Momentum

The total momentum of a system of particles with masses m1,m2,...mn that interact without the action of external forces, is conserved. It means that, being
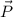`\vec P` and
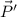`\vec P'` the initial and final momentums respectively:

`\vec P = \vec P'`

Or equivalently, for a two-mass system

`m_1\vec v_1+m_2\vec v_2=m_1\vec v_1'+m_2\vec v_2'`

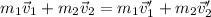
All the velocities are vectors. Let's express the velocities in magnitude v and direction
`\theta` as
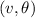`(v,\theta)`. It's rectangular components will be

`v_x=vcos\theta`

`v_y=vsin\theta`

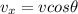
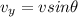
The first car is moving east at 21.2 m/s. Its velocity is

`\vec v_1=(21.2,0^o)`

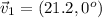
Recall that East is the zero-degree reference for angles

Expressing in rectangular form:

`\vec v_1=<21.2cos0^o,21.2sin0^o>=<21.2,0>`

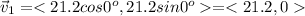
The second car is moving south at 17.5 m/s. Its velocity is

`\vec v_2=(17.5,270^o)`

`\vec v_2=<17.5cos270^o,17.5sin270^o>=<0,-17.5>`

The total initial momentum is

`\vec P=m_1\vec v_1+m_2\vec v_2`

`\vec P=1445<21.2,0>+2625<0,-17.5>`

`\vec P=<30634,-45937.5>\ Kg.m/s`

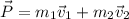
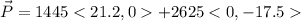
They collide and stick together in a common mass and velocity \vec v', thus

`\vec P'=(m_1+m_2)\vec v'`

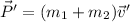
It must be equal to the initial momentum, thus

`(m_1+m_2)\vec v'=<30634,-45937.5>\ Kg.m/s`

Solving for
`\vec v'`

`\displaystyle \vec v'=(<30634,-45937.5>\ Kg.m/s)/(m_1+m_2)`

`\displaystyle \vec v'=(<30634,-45937.5>\ Kg.m/s)/(4070)`

`\displaystyle \vec v'=<7.53,-11.29>\ m/s`

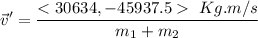
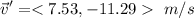
The magnitude of \vec v' is

`|\vec v'|=√(7.53^2+(-11.29)^2)=13.57\ m/s`

The direction angle is

`\displaystyle tan\theta=(-11.29)/(7.53)`

`\displaystyle \theta=-56.30^o`

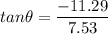
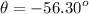
After the collision, they move with a speed of 13.57 m/s at 56.30° south of east