Question

Find the equation for the circle with a diameter whose endpoints are (3,-1) and (-2,-1)

Answer 1

The equation for the circle is:
`(x-(1)/(2) )^2 + y^2 = ((5)/(2) )^2`

Explanation:

Here, let us assume the two end points of the diameter are:

P (3,-1) and Q (-2,-1)

Now, as we knew diameter is the chord that passer through the center of the circle.

⇒ Mid point of DIAMETER = Center coordinates of the Circle

Let us assume O(x,y) is the center of the line segment PQ.

So, by MID POINT FORMULA:

`(x,y)= ((3 -2)/(2)  , (-1-1)/(2) )  =( (1)/(2),(-2)/(2))  \\\implies (x,y) =( 0.5  , -1)`

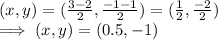
⇒ The center coordinates of the circle = O(0.5,-1) ...... (1)

Now, RADIUS = Half of DIAMETER

Using DISTANCE FORMULA:

`PQ  = √((3-(-2))^2 + (-1 - (-1))^2)   = √((5)^2 + 0)  = 5`

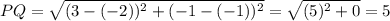
So, Radius = 5/2 = 2.5 units

Now, the equation of circle with radius r and center coordinate ( h,k) is given as:
`(x-h)^2 + (y-k)^2 = r^2`

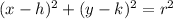
Substitute r = 2.5 and (h,k) = (0.5,0) we get:

`(x-0.5)^2 + (y-0)^2 = (2.5)^2\\\implies (x-(1)/(2) )^2 + y^2 = ((5)/(2) )^2`

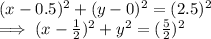
Hence the equation for the circle is:
`(x-(1)/(2) )^2 + y^2 = ((5)/(2) )^2`