Answer:
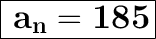
Explanation:
Consider the following sequence as an arithemetic progression (AP). Here, we need to find the 60th term of the given AP.
According to the AP,
- First term (a) = 8
- Common difference (d) =
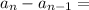 8 - 5 = 3
8 - 5 = 3 - Number of terms (n) = 60
- Last term
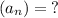
Now, use the formula →
 & substitute the values in it to find the value of '
& substitute the values in it to find the value of '
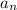 '.
'.
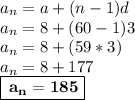
The value of '
 ' in the given AP is 185.
' in the given AP is 185.
