Answer:
a) 68%.
b) 93.3%
c) 61.7%
Explanation:
We are asked to find the percentage of data under normal distribution for given boundaries.
a) The percentage of data that are within 1 standard deviation of the mean.
We will use empirical rule to solve our given problem.
Empirical rule states that approximately 68% of the data lies within one standard deviation of the mean, therefore, answer for part (a) would be 68%.
b) Since z-score represents that a data point is how many standard deviation above or below mean.
We need to find
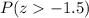 . We will use formula
. We will use formula
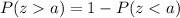 to solve our given problem.
to solve our given problem.
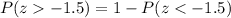
Using normal distribution table, we will get:

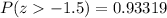
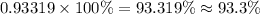
Therefore, approximately 93.3% of the data is to the right of 1.5 standard deviations below the mean.
c) We need to find
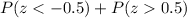 .
.
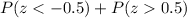
Since normal distribution is symmetric so both these values will be equal.
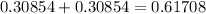
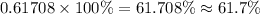
Therefore, approximately 61.7% of the data set is more than 0.5 standard deviations away from the mean.