Answer:
The correct option is D. 13.0 unit
Therefore the Radius of a Circle is 13.0 unit (nearest tenth of a unit)
Explanation:
Given:
Let C be the center of a Circle
C = (x₁ , y₁) = ( 5 , 4)
Let A be the point on a Circle
A = (x₂ , y₂) = ( 12 , 15)
To Find:
Radius, CA = ?
Solution:
Radius :
The radius of a circle is the distance from the center of the circle to any point on its Circle.
So By Distance Formula Between Two point is given as
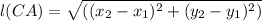
Substituting the values we get


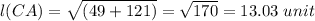
Therefore the Radius of a Circle is 13.0 unit