Question

Given that DF/PR=FE/RQ=3/2, what additional information is needed to prove △DEF ~ △PQR using the SSS similarity theorem?

A. DE ≅ PQ

B.
C. DE/EF = 3/2

D. DE/PQ = 3/2

Answer 1

D.
`(DE)/(PQ) = (3)/(2)`

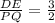
Explanation:

Given:

`(DE)/(PR)=(FE)/(RQ) = (3)/(2)`

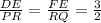
SSS Similarity theorem: Triangles are similar if all three sides in one triangle are in the same proportion to the corresponding sides in the other.

Applying SSS theorem to ΔDEF and Δ PQR :

`(DE)/(PQ) = (EF)/(QR) = (DF)/(PR)`

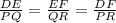
But

`(DF)/(PR) = (EF)/(RQ) = (3)/(2)`

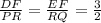
Therefore,

`(DE)/(PQ) = (3)/(2)`

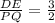
This is the additional information needed to show the triangles are similar as per SSS similarity theorem.