Question

Suppose that A2 = 0 for some matrix A. Prove that the only possible eigenvalues of A are then 0.

Answer 1

The eigenvector of A is not equal to zero, then we can say λ
`^(2)` or λ = 0. Therefore, the only possible eigenvalues of A are 0.

Explanation:

If we assume that λ is the eigenvalue of the matrix A and the eigenvector of the matrix A is ⁻ˣ. Therefore:

For
`A^(2) = 0`

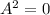
we have:

⁻0 = [⁰₀⁰₀][⁻ˣ] =
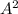`A^(2)`*[⁻ˣ] = Aλ[⁻ˣ] = λ
`^(2)`[⁻ˣ]

In the expression above, ⁻ˣ is not equal to zero, then λ
`^(2)` = 0 or λ is = 0. This shows that the only possible eigenvalues of A are zero '0'