Answer:
Sum of the power dissipated by each resistor connected in parallel.
Solution:
As per the question:
Let the equal value resistor be of R'
 each.
each.
In parallel, the equivalent resistance is given by:
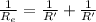
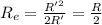
The power dissipated in parallel is given by:
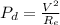
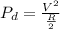
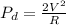 (1)
(1)
Also, it can be seen that the overall power dissipated in the resistors is equal to the sum of the power dissipated in each resistor.
Now,
Power dissipated in one resistor, P =
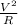
Sum of the power dissipated in each resistor, P =
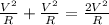 (2)
(2)
Thus from eqn (1) and (2), we can say that the sum of the power in the two resistors connected in parallel is equal.