Question

Refer to the diagram below. Surveyors know that ΔPQR and ΔSTR are similar. What is PQ, the distance across the lake?

Answer 1

Incomplete Question the Complete Question is here

Refer to the diagram below. Surveyors know that ∆PQR and ∆STR are similar. What is PQ, the distance across the lake?

3.20 km

3.60 km

2.80 km​

3.24 km

Answer:

The Last option is correct 3.24 km

Therefore the distance across the lake is PQ = 3.24 km.

Explanation:

Given:

ΔPQR and ΔSTR are Similar

ST = 1.80 km

TR = 1.25 km

QR = 2.25 km

To Find:

Distance across the lake, PQ = ?

Solution:

ΔPQR ~ ΔSTR ..........Given:

If two triangles are similar then their sides are in proportion.

`(PQ)/(ST) =(QR)/(TR) \textrm{corresponding sides of similar triangles are in proportion}\\`

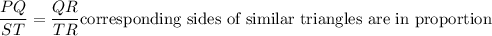
Substituting the values we get

`(PQ)/(1.80) =(2.25)/(1.25)\\\\PQ=(4.05)/(1.25)=3.24\ km\\\therefore PQ = 3.24\ km`

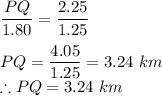
Therefore the distance across the lake is PQ = 3.24 km.