Question

Analyze a graph In Exercise,analyze and sketch the graph of the function.Label any relative extrema, points of inflation,and asymptotes.

f(x) = e^x - e^-x/2

Answer 1

Explanation:

Given is a function f(x)

`f(x) = e^x -e^{-\frac{{x}}{2}`

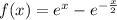
We have to analyse and sketch the graph

X intercept : Put y =0, we get x=0

Y intercept: Put x =0 , we get y =0

No exception for x or y and hence set of all real numbers is domain and range.

`f'(x) = (e^x +1/2 e^{-\frac{{x}}{2})`

f' becomes 0 not at finite points

So relative extreme

Graph is sketched and attached