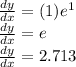Answer:
Equation of tangent:
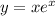
At point (1,0):
y = 2.713
Explanation:
The equation of tangent line to the function can be calculated by taking the first derivative.
We have,
![y = xe^(x)-e^(x)\\(dy)/(dx)=(d)/(dx)[ xe^(x) ]-(d)/(dx) [e^(x)]\\](https://img.qammunity.org/2021/formulas/mathematics/college/lpyge9rjhccgemp8n8adb6g3bazm305buj.png)
Applying Product Rule:
d/dx [u.v] = (d/dx u) . (v) + (u) . (d/dx v)
Therefore,
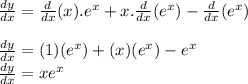
The above equation is the equation of tangent line.
The point given is (1,0):
So,