Question

Use integration by parts to find the integrals in Exercise.
∫^3_0 3-x/3e^x dx.

Answer 1

8.733046.

Explanation:

We have been given a definite integral
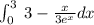`\int _0^3\:3-(x)/(3e^x)dx`. We are asked to find the value of the given integral using integration by parts.

Using sum rule of integrals, we will get:

`\int _0^3\:3dx-\int _0^3(x)/(3e^x)dx`

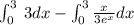
We will use Integration by parts formula to solve our given problem.

`\int\ vdv=uv-\int\ vdu`

Let
`u=x` and
`v'=(1)/(e^x)`.

Now, we need to find du and v using these values as shown below:

`(du)/(dx)=(d)/(dx)(x)`

`(du)/(dx)=1`

`du=1dx`

`du=dx`

`v'=(1)/(e^x)`

`v=-(1)/(e^x)`

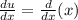
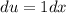
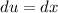
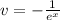
Substituting our given values in integration by parts formula, we will get:

`(1)/(3)\int _0^3(x)/(e^x)dx=(1)/(3)(x*(-(1)/(e^x))-\int _0^3(-(1)/(e^x))dx)`

`(1)/(3)\int _0^3(x)/(e^x)dx=(1)/(3)(-(x)/(e^x)- ((1)/(e^x)))`

`\int _0^3\:3dx-\int _0^3(x)/(3e^x)dx=3x-(1)/(3)(-(x)/(e^x)- ((1)/(e^x)))`

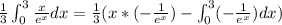
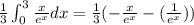
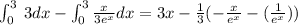
Compute the boundaries:

`3(3)-(1)/(3)(-(3)/(e^3)- ((1)/(e^3)))=9+(4)/(3e^3)=9.06638`

`3(0)-(1)/(3)(-(0)/(e^0)- ((1)/(e^0)))=0-(-(1)/(3))=(1)/(3)`

`9.06638-(1)/(3)=8.733046`

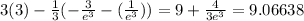
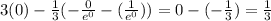
Therefore, the value of the given integral would be 8.733046.