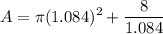Answer:
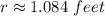
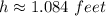
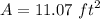
Explanation:
Optimizing With Derivatives
The procedure to optimize a function (find its maximum or minimum) consists in :
- Produce a function which depends on only one variable
- Compute the first derivative and set it equal to 0
- Find the values for the variable, called critical points
- Compute the second derivative
- Evaluate the second derivative in the critical points. If it results positive, the critical point is a minimum, if it's negative, the critical point is a maximum
We know a cylinder has a volume of 4
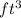 . The volume of a cylinder is given by
. The volume of a cylinder is given by
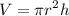
Equating it to 4
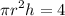
Let's solve for h
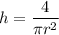
A cylinder with an open-top has only one circle as the shape of the lid and has a lateral area computed as a rectangle of height h and base equal to the length of a circle. Thus, the total area of the material to make the cylinder is
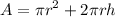
Replacing the formula of h
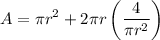
Simplifying
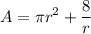
We have the function of the area in terms of one variable. Now we compute the first derivative and equal it to zero
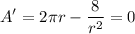
Rearranging
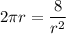
Solving for r
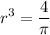
![\displaystyle r=\sqrt[3]{(4)/(\pi )}\approx 1.084\ feet](https://img.qammunity.org/2021/formulas/mathematics/high-school/jfh4ccjug7xkp8xmh8a1vb9grgydqjlp4g.png)
Computing h
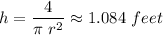
We can see the height and the radius are of the same size. We check if the critical point is a maximum or a minimum by computing the second derivative

We can see it will be always positive regardless of the value of r (assumed positive too), so the critical point is a minimum.
The minimum area is