Question

Calculate the indefinite integral: (Assume t> a and t > b. Use C for the constant of integration.)

∫ t dt/ (t-a) (t - b) = ___________

Answer 1

`\int \left( (X)/(t-a)+(Y)/(t-b) \right)dt=(1)/((b-a)) \left( -a\cdot log(t-a)+b\cdot log(t-a)\right) + C`

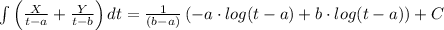
Step-by-step explanation:

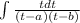`\int (tdt)/((t-a)(t-b))` (1)

We can use partial fraction to solve this kind of integral.

In partial fraction we need to split the this fraction in two new fractions:

`(t)/((t-a)(t-b))=(X)/(t-a)+(Y)/(t-b)` (2)

Now, we need to find the values of X and Y.

Let's work the right equation of (2). We can use least common denominator

`(X)/(t-a)+(Y)/(t-b)=(X(t-b)+Y(t-a))/((t-a)(t-b))=(Xt-Xb+Yt-Ya)/((t-a)(t-b))`

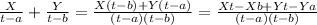
Now we can use common factor in the numerator.

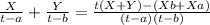`(X)/(t-a)+(Y)/(t-b)=(t(X+Y)-(Xb+Xa))/((t-a)(t-b))` (3)

If we see, we can compere the (3) whit the left side of (2).

The numerators are the same, so we can compare term by term, like this:

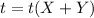`t=t(X+Y)` (4)

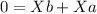`0=Xb+Xa` (5)

We just need to solve the system of equations ((4) and (5)) to find X and Y.

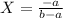`X=(-a)/(b-a)` and
`Y=(b)/(b-a)`

Now, putting this two values in (2) and next we will take the integral.

`\int \left((X)/(t-a)+(Y)/(t-b) \right)=\int \left((-a)/((b-a)(t-a))+(b)/((b-a)(t-b)) \right)=(1)/((b-a)) \int \left((-a)/(t-a)+(b)/(t-a)\right)dt`

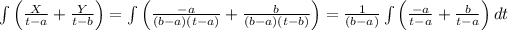
Let's recall that the integral of (1/(x-a))dx is log(x-a), so using it we can find the integral.

`\int \left((X)/(t-a)+(Y)/(t-b) \right)dt=(1)/((b-a))\left( -a\cdot log(t-a)+b\cdot log(t-a)\right) + C`

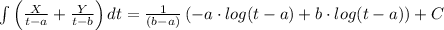
I hope it helps you!