Question

David helped his father stick the pond with baby catfish and blue gill.They out in a total of 75 fish.If the number of blue gill was doubled and the number of catfish tripled,there would be 175 fish in the pond.How many catfish were put in the pond.

Answer 1

By setting up a system of equations based on the given conditions, we calculate that there were 25 catfish originally put into the pond.

Step-by-step explanation:

The problem we're presented with can be modeled using algebra. Let's denote the number of catfish as C and the number of blue gill as B. From the information given:

• The total number of fish originally put in the pond is 75, so C + B = 75.
• If the number of catfish is tripled and the number of blue gill is doubled, the total would be 175 fish. Therefore, 3C + 2B = 175.

We now have a system of linear equations to solve:

1. C + B = 75
2. 3C + 2B = 175

First, solve the first equation for B: B = 75 - C.

Next, substitute B in the second equation:

3C + 2(75-C) = 175

Expand and simplify:

3C + 150 - 2C = 175

C = 175 - 150

C = 25

Therefore, 25 catfish were originally put in the pond.

Answer 2

50 catfish were put in the pond.

Step-by-step explanation:

Given:

Total Number of fishes in the pond = 75

Let number of catfish be 'c'.

Also Let number of blue gill be 'b'

So We can say that;

Total Number of fishes in the pond is equal to sum of number of catfish and number of blue gill.

framing in equation form we get;

`c+b =75 \ \ \ \ equation \ 1`

Also Given:

If the number of blue gill was doubled and the number of catfish tripled,there would be 175 fish in the pond.

It means that;

2 multiplied by number of catfish plus 3 multiplied by number of blue gill is equal to 175.

framing in equation form we get;

`2c+3b =175 \ \ \ \ equation \ 2`

Now Multiplying equation 1 by 2 we get;

`2(c+b) =75*2\\\\2c+2b =150 \ \ \ \ equation\ 3`

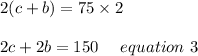
Now Subtracting equation 3 from equation 2 we get;

`(2c+3b)-(2c+2b)=175-150\\\\2c+3b-2c-2b=25\\\\b=25`

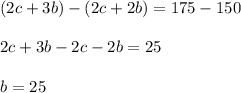
Now Substituting the Value of b in equation 1 we get;

`c+b=75\\\\c+25=75\\\\c=75-25 =50`

Hence 50 catfish were put in the pond.