Answer:
Proof is given below.
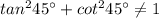
Explanation:
To prove:
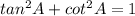 is not a trigonometric identity.
is not a trigonometric identity.
A trigonometric identity is an equation whose left hand side is always to the right hand side for any value of the given angle.
A counterexample is a method used to counter the given statement by taking a random value for the given quantity and disproving the left and right side of the equation.
So, let us take A = 45°
Then, left hand side of the equation becomes;
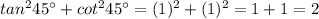
Therefore, the value of left hand side of the equation on plugging in 45° for A gives the result as 2.
But the right hand side of the equation is equal to 1.
Therefore,
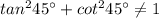
So, this violates the given equation and hence the given equation is not always true. So, it's not a trigonometric identity.