Option D
The regression line predicts that at x = 4, y equals 2143
Solution:
Given that when x equals 4, log(y) will equal 3.331
To find: Find y when x equals 4
Since we have given that,
When x = 4,
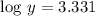
We need to find the value of 'y' when x = 4

Since it is logarithmic function with base 10, Raise to power of 10 on both sides,

On rounding to nearest whole number, we get 2143
Thus Option D is correct