Question

How much would you have to

deposit today to end up with
$1000 in 10 years if yearly
interest is 6% compounded
quarterly?

Answer 1

551.26

Explanation:

I just did it on my calculator so I’m not sure abt the steps

but I hope I helped.

Answer 2

The principal sum to be deposited is $ 551.26

Solution:

Given that,

Amount after 10 years = $ 1000

Rate of Interest = 6 % compounded quarterly

Number of years = 10 years

Principal = ?

The formula for compound interest, including principal sum, is:

`A=p\left(1+(r)/(n)\right)^(n t)`

Where,

A = the future value of the investment/loan, including interest

P = the principal investment amount (the initial deposit or loan amount)

r = the annual interest rate (decimal)

n = the number of times that interest is compounded per unit t

t = the time the money is invested or borrowed for

Since interest is compounded quarterly, n = 4

`r = 6 \% = (6)/(100) = 0.06`

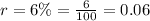
Substituting the values in formula,

`\begin{aligned}&1000=p\left(1+(0.06)/(4)\right)^(4 * 10)\\\\&1000=p(1+0.015)^(40)\\\\&1000=p(1.015)^(40)\\\\&1000=p * 1.8140\\\\&p=(1000)/(1.8140)=551.26\end{aligned}`

Thus the principal sum to be invested is $ 551.26