Question

The line with equation a + 4b = 0 coincides with the terminal side of an angle θ in standard position and cos θ > 0. What is the value of sinθ ?

a. -(√17/17)
b. -(17/√17)
c. -(14/√17)
d. -(17/√14)

Answer 1

Answer: A

Step-by-step explanation: I took the test

Answer 2

Option A. -(
`(√(17))/(17)`)

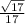Explanation:

Equation of a given line is a + 4b = 0 or b = -
`(1)/(4)a`

This in the form of y = mx + b, which is slope-intercept form.

Here slope of the line is (
`-(1)/(4)`).

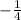Or tanθ =
`((-1))/(4)`

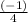
This line coincides with the terminal side of the angle in standard position where cosθ > 0

Since tanθ =
`\frac{\text{Height}}{\text{Base}}`

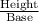
and sinθ =
`\frac{\text{height}}{\txt{Hypotenuse}}`

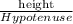
Hypotenuse =
`\sqrt{\text{height}^(2)+\text{Base}^(2)}`

=
`\sqrt{(1^(2)+4^(2))}`

=
`√(17)`

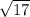
Since tanθ is negative and cosθ > 0 that means θ lie in fourth quadrant.

Therefore, sinθ will be negative.

[
`(-sin\theta)/(+cos\theta)=-tan\theta` ]

sinθ = -
`(1)/(√(17))`

= -
`(1)/(√(17))* (√(17) )/(√(17)) = -(√(17))/(17)`

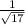
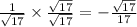
Answer will be option A.