Answer:
90 km/h
Explanation:
Given: Joe´s speed= 60km/h
Dave´s speed= 70km/h
Now, we need to use vector theory to find the rate at which the distance between the cars decreasing when Joe's car is 0.4 km and Dave's is 0.3 km from the intersection.
∴ Let the intersection be the origin and time (t) will be 0 .
And let the distance between the car be "s"
We know, distance (d)= speed (s)\time (t)
∴ Joe´s distance
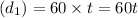
Dave´s distance
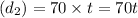
Now, Joe´s change in position is Vector=
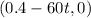
Dave´s change in position is Vector=

Next, find the distance between the cars
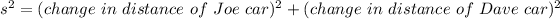
⇒
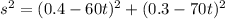
⇒
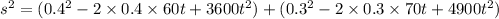
⇒
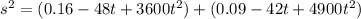
Opening parenthesis
⇒
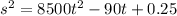 --- equation (1)
--- equation (1)
We know, t=0 at the point of intersection of two roads.
∴
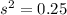
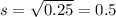
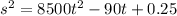 --- equation (1)
--- equation (1)
Using differentiation, to differetiate the distance with respect to t.
⇒
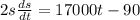
We know, t=0 at the point of intersection of two roads.
∴
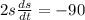
cross multiplying
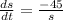
Now, subitituting the value of s in the equation.
∴
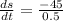
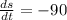
Hence, at 90km/h rate is the distance between the cars decreasing when Joe's car is 0.4 km and Dave's is 0.3 km from the intersection.