Answer:
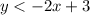
Explanation:
First, we use the given points to find the slope of such line.
The formula to find the slope is
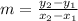
In this case, the points are (0,3) and (2,-1).

Then, we use the point-slope formula to find the equation
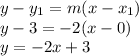
Now, notice that everything to the left of the line is shaded, that means the origin must be part of the solutions.
Therefore, the right inequality is
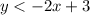
(The image attached shows the graph)