Answer:
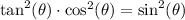
Explanation:
We have been given a trigonometric identity
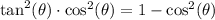 . We are asked to determine the first step of the proof.
. We are asked to determine the first step of the proof.
We will use identity
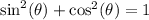 to prove our given identity.
to prove our given identity.
From above identity, we will get:
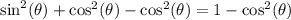
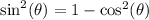
So, we will substitute
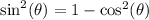 is our given identity as:
is our given identity as:
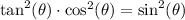
Therefore, the first line of the proof would be
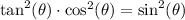 .
.
Upon dividing both sides of equation by
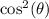 , we will get:
, we will get:
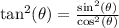

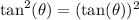

Hence proved.