Question

The statistical difference between a process operating at a 5 sigma level and a process operating at a 6 sigma level is markedly different when it comes to the number of defects present

Answer 1

True

Explanation:

A six sigma level has a lower and upper specification limits between
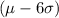`\\ (\mu - 6\sigma)` and
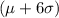`\\ (\mu + 6\sigma)`. It means that the probability of finding no defects in a process is, considering 12 significant figures, for values symmetrically covered for standard deviations from the mean of a normal distribution:

`\\ p = F(\mu + 6\sigma) - F(\mu - 6\sigma) = 0.999999998027`

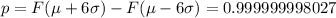
For those with defects operating at a 6 sigma level, the probability is:

`\\ 1 - p = 1 - 0.999999998027 = 0.000000001973`

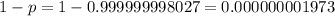
Similarly, for finding no defects in a 5 sigma level, we have:

`\\ p = F(\mu + 5\sigma) - F(\mu - 5\sigma) = 0.999999426697`.

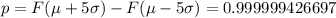The probability of defects is:

`\\ 1 - p = 1 - 0.999999426697 = 0.000000573303`

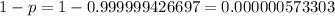
Well, the defects present in a six sigma level and a five sigma level are, respectively:

`\\ {6\sigma} = 0.000000001973 = 1.973 * 10^(-9) \approx (2)/(10^9) \approx (2)/(1000000000)`

`\\ {5\sigma} = 0.000000573303 = 5.73303 * 10^(-7) \approx (6)/(10^7) \approx (6)/(10000000)`

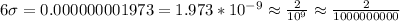
Then, comparing both fractions, we can confirm that a 6 sigma level is markedly different when it comes to the number of defects present:

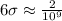`\\ {6\sigma} \approx (2)/(10^9)` [1]

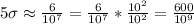`\\ {5\sigma} \approx (6)/(10^7) = (6)/(10^7)*(10^2)/(10^2)=(600)/(10^9)` [2]

Comparing [1] and [2], a six sigma process has 2 defects per billion opportunities, whereas a five sigma process has 600 defects per billion opportunities.