Answer:
a). Graph attached
b). 20 ft
c). 20 ft
d). 1 : 1
e). 28.28 feet
f). 5.66 feet per second
g). (0, 0)
Explanation:
A robot crosses (10, 10) at 1 second and (30, 30) at 6 seconds with a constant speed.
a). As shown in the figure attached.
b). Change in x-coordinates = 30 - 10 = 20 feet
c). Change in the y-coordinates = 30 - 10 = 20 feet
d). Ratio of change in y to change in x coordinates =

= 1 : 1
e). Distance traveled by robot between these points =
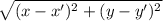
=
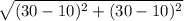
=
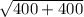
=
 feet
feet
= 28.28 ft
f). Speed of the robot =
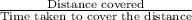
=

=
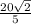
=
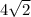
= 5.66 feet per second
g). Robot stared from the origin (0, 0).