Answer:
1.
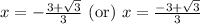
2. Quadratic formula is the most efficient way to solve this equation.
Explanation:
We have been given an equation
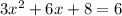 . We are asked to solve our given equation using quadratic formula.
. We are asked to solve our given equation using quadratic formula.
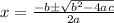 , where,
, where,
b = Coefficient of x term,
c = Constant,
a = Coefficient of
 term.
term.
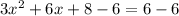
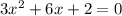
Upon substituting our given values, we will get:
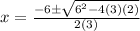

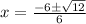
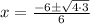
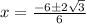
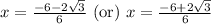
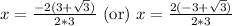
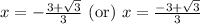
Therefore, the solutions for our given equation are
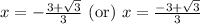 .
.
2. We cannot factor our given equation by splitting the middle term because there are no such numbers which add up-to 6 and whose product is 6.
Therefore, the quadratic formula is the most efficient way to solve this equation.