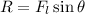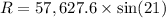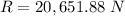Answer:
Step-by-step explanation:
Given
Weight of helicopter
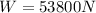
inclination of blades with vertical
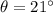
Suppose
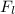 is the lifting Force
is the lifting Force
Now using F-B-D
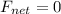 as helicopter is moving with constant velocity
as helicopter is moving with constant velocity
balancing forces in Vertical Force
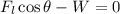
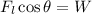
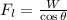
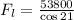
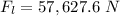
Now sin component of lift is air resistance

where R=air resistance