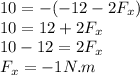Question

A particle moves through an xyz coordinate system while a force acts on it. When the particle has the position vector r with arrow = (2.00 m)i hat − (3.00 m)j + (2.00 m)k, the force is F with arrow = Fxi hat + (7.00 N)j − (5.00 N)k and the corresponding torque about the origin is vector tau = (1.00 N · m)i hat + (0.400 N · m)j + (−0.400 N · m)k. Determine Fx. -I know that you have to find the vector components of F x r, and I did for j and k since i cancels out. But I keep getting the wrong answer. Please provide the solution and the correct answer. I only have three attempts left.

Answer 1

The question is incomplete, below is the complete question "A particle moves through an xyz coordinate system while a force acts on it. When the particle has the position vector r with arrow = (2.00 m)i hat − (3.00 m)j + (2.00 m)k, the force is F with arrow = Fxi hat + (7.00 N)j − (5.00 N)k and the corresponding torque about the origin is vector tau = (4 N · m)i hat + (10 N · m)j + (11N · m)k.

Determine Fx."

`F_(x)=-1N.m`

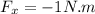
Step-by-step explanation:

We asked to determine the "x" component of the applied force. To do this, we need to write out the expression for the torque in the in vector representation.

torque=cross product of force and position . mathematically this can be express as

`T=r*F`

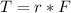
Where

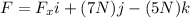`F=F_(x)i+(7N)j-(5N)k` and the position vector

`r=(2m)i-(3m)j+(2m)k`

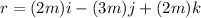
using the determinant method to expand the cross product in order to determine the torque we have

`\left[\begin{array}{ccc}i&j&k\\2&-3&2\\ F_(x) &7&-5\end{array}\right]\\\\`

by expanding we arrive at

`T=(18-14)i-(-12-2F_(x))j+(12+3F_(x))k\\T=4i-(-12-2F_(x))j+(12+3F_(x))k\\\\`

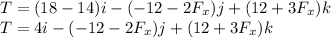
since we have determine the vector value of the toque, we now compare with the torque value given in the question

`(4Nm)i+(10Nm)j+(11Nm)k=4i-(-12-2F_(x))j+(12+3F_(x))k\\`

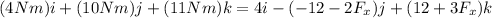
if we directly compare the j coordinate we have

`10=-(-12-2F_(x))\\10=12+2F_(x)\\ 10-12=2F_(x)\\ F_(x)=-1N.m`