Question

Two steel guitar strings have the

samelength. String A has a diameter of 0.50mm and is under 440.0 N
oftension. String B has a diameter of 1.0 mm and is under a
tensionof 820.0 N. Find the ratio of the wave speeds, Va/Vb, in
these twostrings.

Answer 1

`(v_A)/(v_B)=1.47`

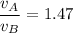
Step-by-step explanation:

given,

diameter of string A = 0.5 mm

tension in string A = 440 N

diameter of string B = 1 mm

tension in string B = 820 N

wave speed =

`v = \sqrt{(T)/(M)}`

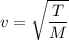
M is the linear density that is mass per unit length =mass / length

mass = density x volume

`M = (\pi r^2d)/(l)`

`v = \sqrt{(T)/(\pi r^2d)}`

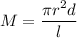
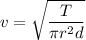
from the above equation

`v \alpha\ \sqrt{(T)/( r^2)}`

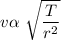
now,

`(v_A)/(v_B)=\sqrt{(T_A)/( T_B)* (r_B^2)/(r_A^2)}`

`(v_A)/(v_B)=\sqrt{(440)/(820)* (1^2)/(0.5^2)}`

`(v_A)/(v_B)=1.47`

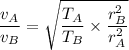
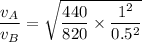
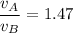
ratio of the wave speed Va/Vb is 1.47