Answer:
H / R = 2/3
Step-by-step explanation:
Let's work this problem with the concepts of energy conservation. Let's start with point P, which we work as a particle.
Initial. Lowest point
Em₀ = K = 1/2 m v²
Final. In the sought height
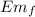 = U = mg h
= U = mg h
Energy is conserved
Em₀ =
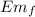
½ m v² = m g h
v² = 2 gh
Now let's work with the tire that is a cylinder with the axis of rotation in its center of mass
Initial. Lower
Em₀ = K = ½ I w²
Final. Heights sought
Emf = U = m g R
Em₀ =
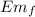
½ I w² = m g R
The moment of inertial of a cylinder is
I =
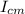 + ½ m R²
+ ½ m R²
I= ½
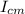 + ½ m R²
+ ½ m R²
Linear and rotational speed are related
v = w / R
w = v / R
We replace
½
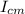 w² + ½ m R² w² = m g R
w² + ½ m R² w² = m g R
moment of inertia of the center of mass
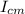 = ½ m R²
= ½ m R²
½ ½ m R² (v²/R²) + ½ m v² = m gR
m v² ( ¼ + ½ ) = m g R
v² = 4/3 g R
As they indicate that the linear velocity of the two points is equal, we equate the two equations
2 g H = 4/3 g R
H / R = 2/3