Answer:
The point of intersection of given polynomials is ( - 5 , - 1 ) .
Explanation:
Given as :
The two polynomials are
y =
 x + 1 ........A
x + 1 ........A
y =
 x - 3 ........B
x - 3 ........B
Let The point of intersection = p = x,y
Now, Solving the equation A and B
So, Putting the value of y from Eq A into Eq B
i.e
 x + 1 =
x + 1 =
 x - 3
x - 3
Rearranging the equation
Or,
 x - (
x - (
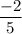 x) = - 3 - 1
x) = - 3 - 1
Or,
 x +
x +
 x = - 3 - 1
x = - 3 - 1
Or, (
 +
+
 ) x = - 4
) x = - 4
Or, (
 ) x = - 4
) x = - 4
Or, (
 ) x = - 4
) x = - 4
∴ x =
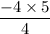
i.e x = - 5
So The value of x = - 5
now put the vale of x into eq B
∵y =
 x - 3
x - 3
So, y =
 × (-5) - 3
× (-5) - 3
Or, y =
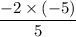 - 3
- 3
Or, y = 2 - 3
i.e y = - 1
So The value of y = - 1
So,The point of intersection = p = x , y = - 5 , - 1
Hence, The point of intersection of given polynomials is ( - 5 , - 1 ) . Answer