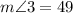Question

Drag and drop an answer to each box to correctly complete the proof.

Given: m∥n , m∠1=50∘ , m∠2=48∘ , and line s bisects ∠ABC .

Prove: m∠3=49∘

Answer 1

The complete demonstration would be

We know that line m and line n are parallel, also
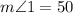`m\angle1=50` and
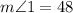`m\angle1=48`, line s bisects
`\angle ABC`.

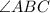Now, by angle addition postulate we know that
`\angle`DEF=
`m\angle1+m\angle2=50+48=98`

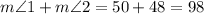
Then, by alternate exterior angle
`\angle`DEF
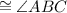`\cong \angle ABC`, because alternate exterior angles are always congruent.

So, by definition of bisector Angles 4 and 5 are congruent, because a bisector line divides the angle in two equal parts.

`m\angle 4=m\angle 5=(98)/(2)=49`

Then, we see that angle 3 and angle 4 are vertical angles, and the congruence postulate states that vertical angles are always congruent. So, by substitution we have

`m\angle 3=49`

Answer 2

See the attached picture: