Question

Which procedure must be done first when proving the Pythagorean Theorem with the diagram?

A. Construct an altitude to hypotenuse c and label it CD
B. Find the Corresponding sides of the similar triangles write proportions
C. Substitute the values into the Pythagorean theorem
D. Use the angle angle similar postulate to find similar triangles

Answer 1

The First step is

Construction:

Construct an altitude to hypotenuse c and label it CD.

The Proof is below.

Explanation:

Given:

In triangle ABC, angle C is 90°

AB = c , AC = b , BC = a

Construction:

Construct an altitude to hypotenuse c and label it CD

segment CD is perpendicular to segment AB.

To Prove:

Pythagoras Theorem

`(BC)^(2)=(AB)^(2)+(AC)^(2)`

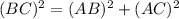
Proof:

In Δ ABC and Δ CBD

∠C ≅ ∠D …………..{ measure of each angle is 90° given }

∠B ≅ ∠B ……….....{Reflexive Property}

Δ ABC ~ Δ CBD ….{Angle-Angle Similarity test}

If two triangles are similar then their sides are in proportion.

`(AB)/(CB) =(BC)/(BD) \textrm{corresponding sides of similar triangles are in proportion}\\`

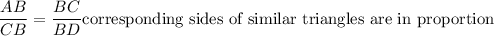
By the cross product property we have

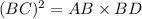`(BC)^(2)=AB* BD` ............( 1 )

Similarly ,

In Δ ABC and Δ ACD

∠C ≅ ∠D …………..{ measure of each angle is 90° given }

∠A ≅ ∠A ……….....{Reflexive Property}

Δ ABC ~ Δ ACD ….{Angle-Angle Similarity test}

If two triangles are similar then their sides are in proportion.

`(AB)/(AC) =(AC)/(AD) \textrm{corresponding sides of similar triangles are in proportion}\\`

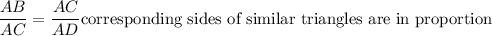
By the cross product property we have

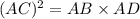`(AC)^(2)=AB* AD`.......... ( 2 )

Now by Adding 1 and 2 we get

`(BC)^(2)+(AC)^(2)=AB* BD+AB* AD=AB(BD+DA)\\\\(BC)^(2)+(AC)^(2)=AB(AB)....A-D-B`

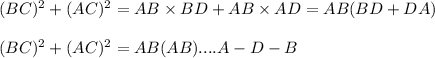
Therefore,

`(BC)^(2)+(AC)^(2)=AB^(2)`

`c^(2)=b^(2)+a^(2)`

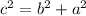
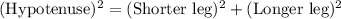`(\textrm{Hypotenuse})^(2) = (\textrm{Shorter leg})^(2)+(\textrm{Longer leg})^(2)` Pythagoras Theorem......Proved