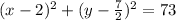Question

The endpoints of a diameter of a circle are (6,2) and (−2,5) . What is the standard form of the equation of this circle?

Answer 1

I took the test...

it's (x-2)^2 + (y-7/2)^2 = 73/4

Answer 2

The standard form of the given circle is

`(x-2)^2+(y-(7)/(2))^2=73`

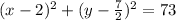
Explanation:

Given that the end points of a diameter of a circle are (6,2) and (-2,5);

Now to find the standard form of the equation of this circle:

The center is (h,k) of the circle is the midpoint of the given diameter

midpoint formula is
`M=((x_(1)+x_(2))/(2),(y_(1)+y_(2))/(2))`

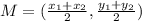
Let
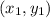`(x_(1),y_(1))` and
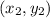`(x_(2),y_(2))` be the given points (6,2) and (-2,5) respectively.

`M=((6-2)/(2),(2+5)/(2))`

`M=((4)/(2),(7)/(2))`

`M=(2,(7)/(2))`

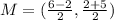
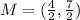
Therefore the center (h,k) is
`(2,(7)/(2))`

now to find the radius:

The diameter is the distance between the given points (6,2) and (-2,5)

`d=\sqrt{(x_(2)-x_(1))^2+(y_(2)-y_(1))^2}`

`=√((-2-6)^2+(5-2)^2)`

`=√((-8)^2+(3)^2)`

`=√(64+9)`

`=√(73)`

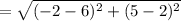
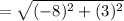
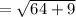
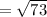
Therefore the radius is
`√(73)`

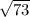
i.e.,
`r=√(73)`

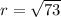
Therefore the standard form of the circle is

`(x-h)^2+(y-k)^2=r^2`

Now substituting the center and radiuswe get

`(x-2)^2+(y-(7)/(2))^2=(√(73))^2`

`(x-2)^2+(y-(7)/(2))^2=73`

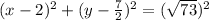
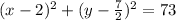
Therefore the standard form of the given circle is

`(x-2)^2+(y-(7)/(2))^2=73`