Answer:
For given equation the value m=6
That is the value of m is 6.
Explanation:
Given equation can be written as
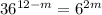
Now to find the value of m in the equation:
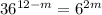
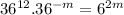 ( using
( using
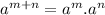 ) Here m=12 and n=-m
) Here m=12 and n=-m
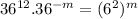 (using
(using
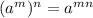 ) Here m=2 and n=m
) Here m=2 and n=m
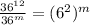 (using the property
(using the property
 Here m=12 and n=m
Here m=12 and n=m
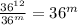
Now multiplying
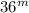 on both sides
on both sides
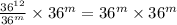
 ( using
( using
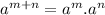 ) Here m=m and n=m
) Here m=m and n=m
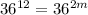
In the above we have base numbers are same so that we can equate the powers of these numbers
12=2m
Rewritting the equation
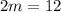
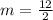
m=6
Therefore the value of m is 6.
For given equation the value m=6.