Question

A geometric sequence is defined by the equation an = (3)3 − n. What are the first three terms of the sequence and what is the common ratio, r?

Answer 1

Question:

A geometric sequence is defined by the equation an = (3)^3 − n. What are the first three terms of the sequence and what is the common ratio, r?

Answer:

The first three terms of sequence is 9, 3 , 1

The common ratio is
`(1)/(3)`

Solution:

The geometric sequence is defined by the equation:

`a_n = (3)^(3-n)`

To find the first three terms of sequence, substitute n = 1, n = 2, n = 3

First term:

Put n = 1 in given equation

`a_1=(3)^(3-1)\\\\a_1 = 3^2\\\\a_1 = 9`

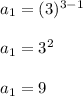
Thus first term of sequence is 9

Second term:

Put n = 2 in given equation

`a_2 = (3)^(3-2)\\\\a_2 = 3^1\\\\a_2 = 3`

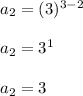
Thus second term of sequence is 3

Third term:

Put n = 3 in given sequence

`a_3 = (3)^(3-3)\\\\a_3=3^0\\\\a_3 = 1`

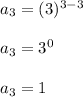
Thus third term of sequence is 1

Thus the first three terms of sequence is 9, 3 , 1

To find common ratio:

Common ratio is found by dividing the two consecutive terms

`a_1 = 9\\\\a_2 = 3\\\\a_3 = 1`

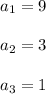
Thus common ratio is obtained as:

`r = (a_2)/(a_1) = (3)/(9) = (1)/(3)`

`r = (a_3)/(a_2)=(1)/(3)`

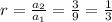
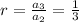
Thus common ratio is
`(1)/(3)`