Answer:
The maximum height 400 feet is attained at t = 5 seconds.
Explanation:
All powers of
 in the equation for
in the equation for
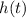 are integers that are greater than or equal to zero. Additionally, the greatest power of
are integers that are greater than or equal to zero. Additionally, the greatest power of
 is two. Hence,
is two. Hence,
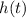 is a quadratic equation about
is a quadratic equation about
 .
.
- Let
 be the coefficient of the
be the coefficient of the
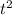 term, and
term, and - Let
 be the coefficient of the
be the coefficient of the
 term.
term.
In this case,
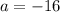 , and
, and
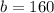 .
.
The question is asking for the maximum value of this equation. Start by finding the
 (time) that would maximize the value of the polynomial.
(time) that would maximize the value of the polynomial.
The graph of a quadratic equation looks like a parabola. Additionally, since the coefficient of
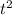 is less than zero, the parabola opens downward. The maximum value of the parabola would be at its vertex. Additionally, at the vertex,
is less than zero, the parabola opens downward. The maximum value of the parabola would be at its vertex. Additionally, at the vertex,
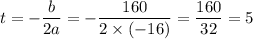 .
.
In other words, the rocket is at its maximum height when time is equal to 5 seconds.
To find that height, let
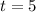 and evaluate
and evaluate
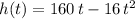 :
:
 .
.
That is: the maximum height of the rocket would be 400 feet.