Question

How many triangles and quadrilaterals altogether can be formed using the vertices of a 7-sided regular polygon?

A. 35
B. 40
C. 50
D. 65
E. 70

Answer 1

Answer: The correct option is

(E) 70.

Step-by-step explanation: We are given to find the number of triangles and quadrilaterals altogether that can be formed using the vertices of a 7-sided regular polygon.

To form a triangle, we need any 3 vertices of the 7-sided regular polygon. So, the number of triangles that can be formed is

`n_t=^7C_3=(7!)/(3!(7-3)!)=(7*6*5*4!)/(3*2*1*4!)=35.`

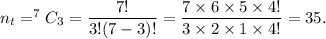
Also, to form a quadrilateral, we need any 4 vertices of the 7-sided regular polygon. So, the number of quadrilateral that can be formed is

`n_q=^7C_4=(7!)/(4!(7-4)!)=(7*6*5*4!)/(4!*3*2*1)=35.`

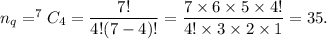
Therefore, the total number of triangles and quadrilaterals is

`n=n_t+n_q=35+35=70.`

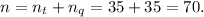
Thus, option (E) is CORRECT.