Answer:
First let's write down the moment of inertia of the objects.
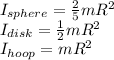
If they all roll without slipping, then the following relation is applied to all ot them:
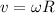
where v is the translational velocity and ω is the rotational velocity.
We will use the conservation of energy, because we know that their initial potential energies are the same. (Here, I will assume that all the objects have the same mass and radius. Otherwise we couldn't determine the difference. )
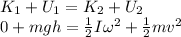
For sphere:
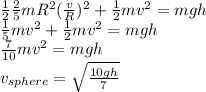
For disk:
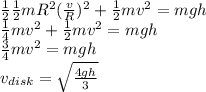
For hoop:

The sphere has the highest velocity, so it arrives the bottom first. Then the disk, and the hoop arrives the last.
Step-by-step explanation:
The moment of inertia can be defined as the resistance to the rotation. If an object has a high moment of inertia, it resist to rotate more so its angular velocity would be lower. In the case of rolling without slipping, the angular velocity and the linear (translational) velocity are related by the radius, so the object with the highest moment of inertia would arrive the bottom the last.