Question

In a study of wait times at an amusement park, the most popular roller coaster has a mean wait time of 17.4 minutes with a standard deviation of 5.2 minutes. If 30 days are randomly selected, find the probability that the mean wait time is greater than 20 minutes.

A: 0.0031

B: 0.1023

C: 0.3207

D: 0.9987

Answer 1

Answer: A: 0.0031

Explanation:

Answer 2

Answer: A: 0.0031

Explanation:

Given : In a study of wait times at an amusement park, the most popular roller coaster has a mean wait time of 17.4 minutes with a standard deviation of 5.2 minutes.

i.e.
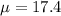`\mu=17.4` and
`\sigma=5.2`

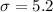
We assume that the wait times are normally distributed.

samples size : n= 30

Let x denotes the sample mean wait time.

Then, the probability that the mean wait time is greater than 20 minutes will be :

`P(x>20)=1-P(x\leq20)\\\\=1-P((x-\mu)/((\sigma)/(√(n)))\leq(20-17.4)/((5.2)/(√(30))))\\\\=1-P(z\leq2.74)\ \ [\because\ z=(x-\mu)/((\sigma)/(√(n)))]\\\\=1-0.9969\ \ [\text{ By z table}]\\\\=0.0031`

Hence, the probability that the mean wait time is greater than 20 minutes.= 0.0031

Thus , the correct answer is A: 0.0031 .