Question

Management at a seaside resort is publishing a brochure and wants to include a statement about the proportion of clear days during their peak season. Out of a random sample of 150 days from over the last two peak seasons, 117 days were recorded as clear. They want to estimate the proportion of clear days to within a 5% margin of error with a 95% confidence interval. What's the sample size necessary to construct this interval?A. 384B. 264C. 383D. 385E. 263

Answer 1

Answer: B. 264

Explanation:

Formula to calculate the sample size 'n' , if the prior estimate of the population proportion (p) is available:

`n= p(1-p)((z)/(E))^2`

, where z = Critical z-value corresponds to the given confidence interval

E= margin of error

Let p be the population proportion of clear days.

As per given , we have

Prior sample size : n= 150

Number of clear days in that sample = 117

Prior estimate of the population proportion of clear days =
`p=(117)/(150)`

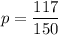
E= 0.05

The critical z-value corresponding to 95% confidence interval = z*= 1.95 (By z-table)

Then, the required sample size will be :

`n= (117)/(150)(1-(117)/(150))((1.96)/(0.05))^2`

Simplify ,

`n= (0.1716)(39.2)^2`

`n= 263.687424\approx264`

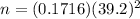
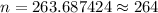
Hence, the sample size necessary to construct this interval =264

Thus the correct option is B. 264