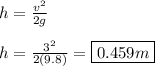Question

A pendulum bob has it maximum speed at 3ms at the lowest position 0. Calculate the height of the bob above 0,where it velocity is 0​

Answer 1

Hello!

We know that at the BOTTOM of the pendulum's trajectory, the bob has a maximum speed. This means that its KINETIC ENERGY is at a maximum, while its Gravitational POTENTIAL ENERGY is at a minimum.

On the other hand, when the bob is at its highest points, the bob has a velocity of 0 m/s, so its KE is at a minimum and its PE is at a maximum.

We can use the work-energy theorem to solve. Let the Initial Energy equal the bob's energy at one of the sides, while the final Energy equals the bob's energy at the bottom.

`E_i = E_f\\\\PE = KE`

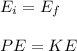
Recall that:
PE = mgh

m = mass (kg)

g = acceleration due to gravity (m/s²)

h = height (m)

KE = 1/2mv²

m = mass (kg)

v = velocity (m/s)

Set the two equal and solve for 'h'.

`mgh = (1)/(2)mv^2`

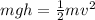
Cancel mass.

`gh = (1)/(2)v^2`

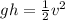
Solve for 'h'.

`h = (v^2)/(2g)\\\\h = (3^2)/(2(9.8)) = \boxed{0.459 m}`