Question

A branch falls from a tree. How fast is the branch moving after 0.28 seconds?

A. 2.7 m/s
B. 1.3 m/s
C. 4.4 m/s
D. 3.1 m/s

Answer 1

A. 2.7 m/s

Step-by-step explanation:

Answer 2

`\boxed {\boxed {\sf A. \ 2.7 \ m/s}}`

Step-by-step explanation:

We want to find how fast a branch is falling after 0.28 seconds.

Essentially, we want to find its final velocity at exactly 0.28 seconds. We will use the following kinematic equation:

`v_f= v_i+at`

The branch fell from the tree, so it initially started at rest or 0 meters per second. The branch is in free fall, so its acceleration is due to gravity, or 9.8 meters per second squared. It falls for 0.28 seconds.

• 
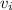  `v_i`= 0 m/s
• a= 9.8 m/s²
• t= 0.28 s

Substitute the values into the formula.

`v_f= 0 \ m/s + (9.8 \ m/s^2)(0.28 \ s)`

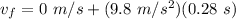
Multiply the numbers in parentheses.

`v_f= 0 \ m/s +(9.8 \ m/s/s * 0.28 \ s )`

`v_f= 0 \ m/s +2.744 \ m/s`

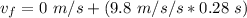
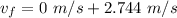
Add.

`v_f= 2.744 \ m/s`

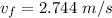
The original measurement of time has 2 significant figures, so our answer must have the same. For the number we found, that is the tenth place. The 4 in the hundredth place tells us to leave the 7.

`v_f \approx 2.7 \ m/s`

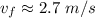
The branch is moving at a velocity of approximately 2.7 meters per second.