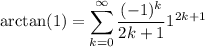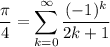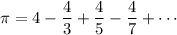Recall that for |x| < 1, we have
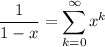
It follows that, for |-x²| = |x|² < 1, or just |x| < 1,
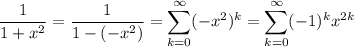
Taking the antiderivative of both sides gives us
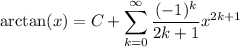
where C is a constant, which we determine to be 0, since taking x = 0 on both sides makes the series vanish, while arctan(0) = 0 since 0 = tan(0). So
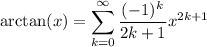
Since tan(π/4) = 1, it follows that π/4 = arctan(1), so in the series we replace x = 1, then solve for π :