Question

Can someone please help me with #1-#5?I don't know how to find the answer.​

Answer 1

Answers:

1. Slope = undefined; y intercept = none
2. Slope = 2; y intercept = -2
3. Slope = -1; y intercept = 1
4. Slope = 0
5. Slope = -4

========================================================

Step-by-step explanation:

1)

For any vertical line, the slope is always undefined. Recall that slope = rise/run. For a vertical line, the run is 0 because there is no left or right movement. This leads to a division by zero error. The vertical line is also parallel to the y axis, meaning that there isn't a y intercept whenever the line isn't directly on top of the y axis.

The equation of this vertical line is x = 3 as all points on this line have the same x coordinate of 3.

-----------------------

2)

We can use the slope formula for the points
`(x_1,y_1) = (-1,-4) \ \text{ and } \ (x_2,y_2) = (3,4)`

So,

`m = (y_2-y_1)/(x_2-x_1)\\\\m = (4-(-4))/(3-(-1))\\\\m = (4+4)/(3+1)\\\\m = (8)/(4)\\\\m = 2\\\\`

The slope is 2.

The y intercept is -2 as this is the location where the diagonal line crosses the y axis.

The equation of this line is y = 2x-2.

-----------------------

3)

Same idea as problem 2.

`(x_1,y_1) = (-2,3)\\\\(x_2,y_2) = (3,-2)\\\\m = (y_2-y_1)/(x_2-x_1)\\\\m = (-2-3)/(3-(-2))\\\\m = (-2-3)/(3+2)\\\\m = (-5)/(5)\\\\m = -1\\\\`

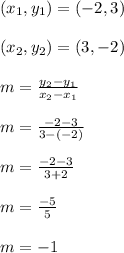
The slope is -1.

The y intercept is 1 because the line cuts through 1 on the y axis.

The equation is y = -1x+1 which is the same as y = -x+1.

-----------------------

4)

Pick any two points from the table. Each column represents a different (x,y) ordered pair point. Those two points selected are then plugged into the slope formula. I'll pick the first two columns.

`(x_1,y_1) = (1,-2)\\\\(x_2,y_2) = (2,-2)\\\\m = (y_2-y_1)/(x_2-x_1)\\\\m = (-2-(-2))/(2-1)\\\\m = (-2+2)/(2-1)\\\\m = (0)/(1)\\\\m = 0\\\\`

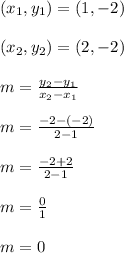
Notice how the y coordinates are the same, which leads to a difference of 0 up top and ultimately the slope itself is also 0. We have a horizontal flat line here. All horizontal lines have a slope of 0.

The y intercept is -2 because all y values for this function are the same.

The equation is y = 0x-2 which is the same as y = -2.

-----------------------

5)

Again I'll pick the first two columns to plug into the slope formula, but you can pick any two columns you prefer.

`(x_1,y_1) = (-3,11)\\\\(x_2,y_2) = (-1,3)\\\\m = (y_2-y_1)/(x_2-x_1)\\\\m = (3-11)/(-1-(-3))\\\\m = (3-11)/(-1+3)\\\\m = (-8)/(2)\\\\m = -4\\\\`

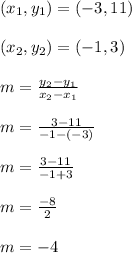
The slope is -4.

Use this slope value, along with any column from the table to form the (x,y) point, to plug into the slope intercept form below. I'll use the first column

y = mx+b

11 = -4*(-3)+b

11 = 12+b

11-12 = b

-1 = b

b = -1

The y intercept is -1.

The equation is y = -4x-1.