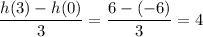Answer:
g, f, h
Explanation:
By definition, the average rate of change of a function f over an interval [a,b] is given by
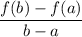
So, in your case, we want to compute the quantity
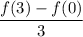
for all the three function
Average rate of change of f:
We will simply use the table to check the values for f(3) and f(0):
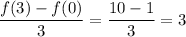
Average rate of change of g:
We will use the graph to to check the values for g(3) and g(0):
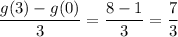
Average rate of change of h:
We can plug the values in the equation to get h(3) and h(0):
h(3)=3^2+3-6=9+3-6=6,
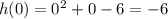
And so the average rate of change is