Question

A cart loaded with bricks has a total mass of 24.7kg and is pulled at constant speed by a rope. The rope is inclined at 27.3° above the horizontal and the cart moves 23.1m on a horizontal floor. The coefficient of kinetic friction is between ground and cart is 0.8.

The acceleration of gravity is 9.8m/s^2.
How much work is done on the cart by the rope?

Answer 1

Approximately
`3.69 * 10^(3)\; \rm J`.

Step-by-step explanation:

Let
`m` denote the mass of this cart of bricks and let
`g` denote the gravitational acceleration. Let
`\mu` denote the constant of kinetic friction between the ground and this cart of bricks.

Refer to the diagram attached. There are four forces on this cart of bricks:

• Weight of the cart and the bricks:
  `W = m \cdot g`.
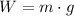• Normal force from the ground:
  `N`.
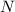• Friction between the cart and the ground:
  `f = \mu \cdot N`.
• Force exerted through the rope,
  `F`.

Consider the force exerted through the rope in two components:

• Horizontal component of
  `F`:
  `F \cos(27.3^(\circ))`.
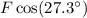• Vertical component of
  `F`:
  `F \sin(27.3^(\circ))`.

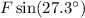Since the velocity of this cart is constant, forces on this cart would be balanced. The following would be equal:

• Vertically, the normal force
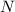  `N` and the vertical component of
  `F` should balance the weight of the cart and the bricks:
  `N + F\, \sin(27.3^(\circ)) = W`.
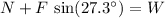• Horizontally, the horizontal component of
  `F` should balance the friction between the cart of bricks and the ground:
  `F\, \cos(27.3^(\circ)) = f`.

However,
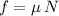`f = \mu \, N`. Thus, the second equation would be equivalent to
`F\, \cos(27.3^(\circ)) = \mu \, N`.

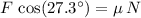The weight of this cart of bricks is
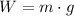`W = m \cdot g`. The first equation would be equivalent to
`N + F\, \sin(27.3^(\circ)) = m \cdot g`.

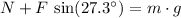The value of
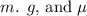`\text{$m$. $g$, and $\mu$}` are all given. Thus, these two equations is a system of two equations for two unknowns,
`\text{$N$ and $F$}`:

`N + F\, \sin(27.3^(\circ)) = m \cdot g`.

`F\, \cos(27.3^(\circ)) = \mu \, N`.

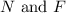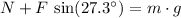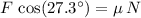Solve this system of equations for
`F`, the size of the force that the rope exerted on the cart or bricks.

Rewrite the first equation to find an expression for
`N`:

`N = m\cdot g - F\, \sin(27.3^(\circ))`.

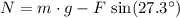Substitute this expression into the second equation:

`F\, \cos(27.3^(\circ)) = \mu\, (m\cdot g - F\, \sin(27.3))`.

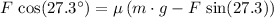Rearrange and solve for
`F`:

`\begin{aligned}F &= (\mu \cdot m \cdot g)/(\cos(27.3^(\circ)) + \sin(27.3^(\circ))) \\ &= (0.8 * 24.7\; \rm kg * 9.81\; \rm m\cdot s^(-2))/(\cos(27.3^(\circ)) + \sin(27.3^(\circ))) \\ &\approx 179.67\; \rm N\end{aligned}`.

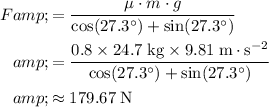Thus, the size of the force that the rope exerted on the cart would be approximately
`179.67\; \rm N`.

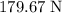The floor is horizontal. Thus, the vertical displacement of this cart of bricks would be
`0`. The vertical component of
`F` would thus have done no work on the cart
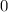`0\!`. The entirety of the work that
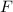`F\!` does on this cart of bricks would come from the horizontal component of this force.

Given that
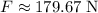`F \approx 179.67\; \rm N`, the horizontal component of this force would be:

`F\, \cos(27.3^(\circ)) \approx 159.66\; \rm N`.

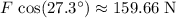The horizontal displacement of this cart of bricks is
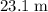`23.1\; \rm m`. Accordingly, the work that the horizontal component of
`F` did on this cart of bricks would be approximately
`159.66\; \rm m * 23.1\; \rm m \approx 3.69 * 10^(3)\; \rm J`.

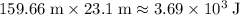Thus, the overall work that this rope did on this cart of bricks would be approximately
`3.69 * 10^(3)\; \rm J`.